Negative

The term "negative," in a mathematical context, refers to real numbers that are less than zero. Negative numbers are the opposite of positive numbers. For example, if zero were to represent a starting point, traveling towards a destination would represent a positive number, while traveling farther away from the destination would represent a negative number. Another common use of negative numbers can be seen with money. Money paid to you is a positive number while money you pay to someone else would be a negative number.

A negative number is indicated using "-" in front of the number. Positive numbers can technically be indicated using a "+", but by convention, positive numbers are written without the plus sign in front of them. Any number without a minus sign is assumed to be positive. Thus, 5 is positive (plus) five, while -5 is negative (minus) five. Typically, the number 0 is considered neither positive nor negative.

Conceptualizing negative numbers

There a number of ways to think about negative numbers. One such way is using a number line. It may be easier to conceptualize negative numbers by seeing them as the counterpart of positive numbers on the other side of zero on the number line:

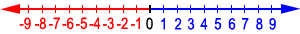
Another way is through using subtraction to get a sense of the relative size of numbers. For example, the problem

12 - 4 = 8

tells us that the difference between 12 and 4 is 8. We can then extend this to the problem

4 - 12 = ?

and conclude that since the difference between 12 and 4 is 8, the difference should remain the same, but negative, since 12 is larger than 4. Thus,

4 - 12 = -8