Perimeter

The perimeter is the total length or distance around a two dimensional shape. In the figure below, the perimeter of each shape is the sum of the lengths of each side, shown in red. The perimeter of a circle or ellipse is called the circumference. For a polygon, the perimeter is the sum of its side lengths.

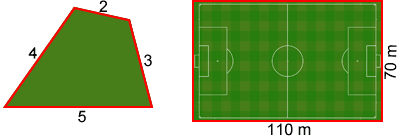
The perimeter of the quadrilateral above is 2 + 3 + 4 + 5 = 14. The perimeter of the soccer field above is 110 + 110 + 70 + 70 = 360 meters. To add a fence around the edges of the entire soccer field, the fence would have to cover 360 meters.

Perimeter of common geometric figures

Below are formulas for the perimeters of some common geometric figures.

Square

The perimeter of a square with side length s is:

P = 4s

Rectangle

The perimeter of a rectangle with length, l, and width, w, is.

P = 2l + 2w

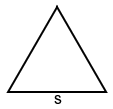
Equilateral triangle

The perimeter of an equilateral triangle with side lengths s is:

P = 3s

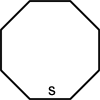
Regular polygon

The perimeter of a regular (all sides are equal) n-sided polygon with side lengths s is:

P = ns

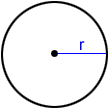
Circle

The circumference of a circle with radius r is:

C = 2πr

Perimeter of similar figures

If geometric figures are similar, the ratio of their perimeters is equal to the ratios of their corresponding sides. For the similar triangles ABC and DEF below,

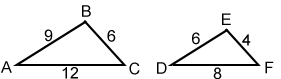
Distance formula

Given the coordinates of the vertices of a polygon in the coordinate plane, the side length of the polygon can be calculated using the distance formula:

where d is the distance between the two points with coordinates (x₁, y₁) and (x₂, y₂). Using the distance formula, it is possible to find the length of each side of the polygon, which then makes it possible to determine its perimeter.