Polyhedron

In Geometry, a polyhedron is a closed space figure whose faces are polygons. The word polyhedron has Greek origins, meaning many faces. The following are a few examples of polyhedra.

Characteristics of a polyhedron

The polygons that form a polyhedron are called faces. The line segments created by two intersecting faces are called edges. The vertices are points where three or more edges meet.

The hexagonal prism above is a polyhedron that has 6 lateral faces that are parallelograms, and 2 faces on the top and bottom, called bases, that are hexagons.

Euler's Theorem

Euler's Theorem shows a relationship between the number of faces, vertices, and edges of a polyhedron. It states that the sum of the faces and vertices minus the number of edges always equals two:

F + V - E = 2

where F is the number of faces, V is the number of vertices, and E is the number of edges of a polyhedron.

Classifications of polyhedra

Polyhedra can be classified in many ways. For example, they can be classified as regular and irregular polyhedra. A regular polyhedron is a polyhedron whose faces are all congruent regular polygons; any polyhedron that does not meet these conditions is considered irregular.

Polyhedra can also be classified as convex and concave. A concave polyhedron has at least one face that is a concave polygon. A polyhedron that is not concave, is convex. Polyhedra can also be classified based on the number of faces it has. For example, a tetrahedron has 4 faces, a pentahedron has 5 faces, and a hexahedron has 6 faces.

The following is a list of terms often used to describe polyhedra based on their characteristics.

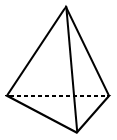
Prisms

Prisms are polyhedra that have two congruent faces, called bases, lying in parallel planes. A prism is typically named by the shape of its polygonal bases. The lateral faces (the sides that are not bases) are parallelograms, rectangles, or squares.

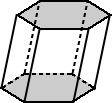
Regular prism	Irregular prism
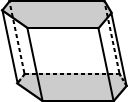The bases for the regular hexagonal prism above have bases that are regular hexagons.	The bases for the hexagonal prism above are irregular hexagon.

Pyramids

Pyramids are polyhedra that has a polygon as its base and triangles as all its other faces. A pyramid is also typically named by the shape of its polygonal base.

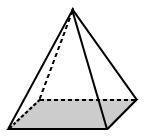
Regular pyramid	Irregular pyramid
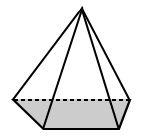The base of the square pyramid above has a base that is a square (a regular polygon).	The base for the trapezoidal pyramid above is a trapezoid with unequal sides (so it is an irregular polygon).

Regular polyhedra

A regular polyhedron is a polyhedron whose faces are all congruent, regular polygons. A regular polyhedron is named based on its number of faces. There are only five polyhedra that are regular polyhedra; these are referred to as Platonic solids.

The five Platonic solids

In the diagram above, each regular polyhedra is named based on its number of faces. The net below each sketch shows a 2D picture of all of the faces of the polyhedron.

Most regular prisms are generally not considered regular polyhedra. A cube is the only regular prism that can also be classified as a regular polyhedron.

Likewise, a regular tetrahedron is the only regular pyramid that is also a regular polyhedron.