Sphere

A sphere is a 3D geometric figure that has a round shape. All points on the surface of the sphere are an equal distance from its center.

Below are some real-life examples of spheres. A basketball, the Earth, and an orange are all examples of geometric figures that have the shape of a sphere.

Properties of a sphere

In Geometry, a sphere is defined as the set of all points in space that are equidistant from a fixed point (the center) of the sphere.

Radius

A radius, r, of a sphere is a line segment from the center of the sphere to any point on the sphere's surface. Three radii (plural for radius) are graphed for the sphere with center at point O below.

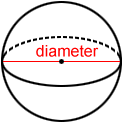
Diameter

Like a circle, a diameter of a sphere is any chord that passes through its center, as shown below.

Great circle

The intersection of a plane and a sphere containing the sphere's center creates a circle. This circle is called a great circle. A plane that intersects a sphere without going through the center will also create a circle. However, it is not a great circle, since a great circle is the largest possible circle that can be created by the intersection of a plane with a sphere. Any other circular intersection has a radius smaller than the great circle.

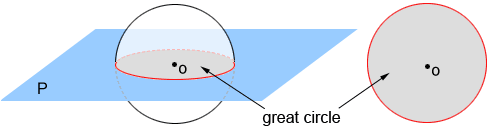
Plane P above intersects sphere O through center O creating a great circle of the sphere, shown in grey. The great circle divides the sphere into two equal hemispheres.

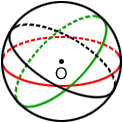
Three great circles in black, red, and green are shown for sphere O above. A sphere contains infinitely many great circles. Each great circle is considered a plane of symmetry since it divides the sphere into two equal parts. This means that a sphere has infinitely many planes of symmetry.

Volume of a sphere

The volume, V, of a sphere is

where r is the radius of the sphere.

Surface area of a sphere

The surface area, S, of a sphere is

S = 4πr²

where r is the radius of the sphere.